New York Lecture: This Tuesday March 19th at Weil Cornell

Talmudology on the Parsha, Pekudei: Happy Pi Day
This weeks parsha, Pekudei, contains additional details about the construction of the Mishkan. We are told how much gold was used:
38:24 שמות
כל־הַזָּהָ֗ב הֶֽעָשׂוּי֙ לַמְּלָאכָ֔ה בְּכֹ֖ל מְלֶ֣אכֶת הַקֹּ֑דֶשׁ וַיְהִ֣י ׀ זְהַ֣ב הַתְּנוּפָ֗ה תֵּ֤שַׁע וְעֶשְׂרִים֙ כִּכָּ֔ר וּשְׁבַ֨ע מֵא֧וֹת וּשְׁלֹשִׁ֛ים שֶׁ֖קֶל בְּשֶׁ֥קֶל הַקֹּֽדֶשׁ׃
All the gold that was applied for the work in all the work of the holy place, even the gold of the offering, was twenty nine talents, and seven hundred and thirty shekels, after the shekel of the sanctuary.
We read how the Ephod, a sort of priestly apron, was made:
39:2 שמות
וַיַּ֖עַשׂ אֶת־הָאֵפֹ֑ד זָהָ֗ב תְּכֵ֧לֶת וְאַרְגָּמָ֛ן וְתוֹלַ֥עַת שָׁנִ֖י וְשֵׁ֥שׁ משְׁזָֽר׃
And he made the efod of gold, blue, and purple, and scarlet, and fine twined linen.
And when it was all finally completed, Moses looked at the finished product, and was pleased. He was very pleased:
39:43 שמות
וַיַּ֨רְא מֹשֶׁ֜ה אֶת־כל־הַמְּלָאכָ֗ה וְהִנֵּה֙ עָשׂ֣וּ אֹתָ֔הּ כַּאֲשֶׁ֛ר צִוָּ֥ה יְהֹוָ֖ה כֵּ֣ן עָשׂ֑וּ וַיְבָ֥רֶךְ אֹתָ֖ם מֹשֶֽׁה׃ {פ}
And Moses saw all the work, and, behold, they had done it as the Lord had commanded, even so had they done it: and Moses blessed them.
The haftarah for this week’s reading is from The First Book of Kings (מלכים א 7:51-8:21) and echoes the theme of the parsha. It describes the completion of the first Temple, which was built by King Solomon. Here is the opening verse:
וַתִּשְׁלַם֙ כל־הַמְּלָאכָ֔ה אֲשֶׁ֥ר עָשָׂ֛ה הַמֶּ֥לֶךְ שְׁלֹמֹ֖ה בֵּ֣ית יְהֹוָ֑ה וַיָּבֵ֨א שְׁלֹמֹ֜ה אֶת־קדְשֵׁ֣י ׀ דָּוִ֣ד אָבִ֗יו אֶת־הַכֶּ֤סֶף וְאֶת־הַזָּהָב֙ וְאֶת־הַכֵּלִ֔ים נָתַ֕ן בְּאֹצְר֖וֹת בֵּ֥ית ה׳
So was ended all the work that King Solomon made for the house of the Lord. And Solomon brought in the things which David his father had dedicated; the silver, and the gold, and the vessels, he did put in the treasuries of the house of the Lord…
In keeping with the topic of the Temple and its vessels, and noting today’s date, we will focus on another special piece of equipment in Solomon’s Temple. It was a large round bowl, and it is mentioned in the same chapter as the haftarah. Read it carefully, then answer this question: What is the value of pi that the verse describes?
מלכים א פרק ז פסוק כג
ויעש את הים מוצק עשר באמה משפתו עד שפתו עגל סביב וחמש באמה קומתו וקוה שלשים באמה יסב אתו סביב
And he made a molten sea, ten amot from one brim to the other: it was round, and its height was five amot, and a circumference of thirty amot circled it.
Answer: THREE.
The circumference was 30 amot and the diameter was 10 amot. Since pi is the ratio of the circumference of a circle to its diameter, pi in the Book of Kings is 30/10=3. Three - no more and no less. Which brings us to today’s date and the math that is celebrated on it.
WHAT IS PI DAY, AND WHEN IS IT CELEBRATED?
From here.
Today, March 14, is celebrated as Pi Day by some of the mathematically inclined in the US. Why? Well, in most of the world, the date is written as day/month/year. So in Israel, all of Europe, Australia, South America and China, today's date, March 14th, would be written as 14/3.
But not here in the US. Here, we write the date as month/day/year; it's a uniquely American way of doing things. (Like apple pie. And guns.) So today's date is 3/14. Which just happen to be the first few digits of pi, the ratio of the circumference of a circle to its diameter.
And that's why each year, some (particularly geeky) Americans celebrate Pi Day on March 14 (3/14). The year 2015 was more Pi'ish than all others, since the entire date (when written the way we do in the US, 3/14/15) reflects five digits of pi, and not just the first three: 31415. Actually we got even more geeky: This day in 2015 at 9:26 and 53 seconds in the morning, the date and time, when written out, represented the first ten digits of Pi: 3141592653.
So that's why Pi Day is celebrated here in the US - and probably not anywhere else. (It has even been recognized as such by a US Congressional Resolution. Really. I'm not making this up. And who says Congress doesn't get anything done?)
Pi in the Bible
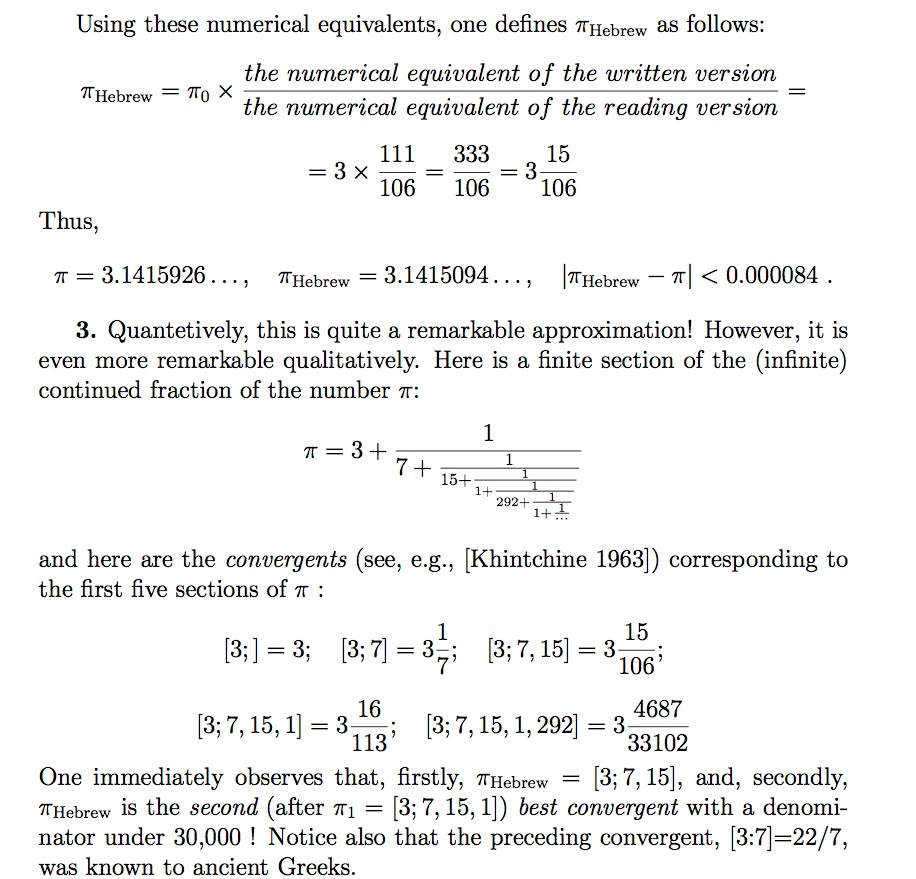
We have just seen how the value of pi that we would derive from the bowl in Solomon’s Temple is three (and not 3.1415….). There are lots of papers on the value of pi in the the Bible. Many of them mention an observation that seems to have been incorrectly attributed to the Vilna Gaon. The verse we cited from מלאכים א׳ spells the word for line as קוה, but it is pronounced as though it were written קו. (In דברי הימים ב׳ (II Chronicles 4:2) the identical verse spells the word for line as קו.) The ratio of the numerical value (gematria) of the written word (כתיב) to the pronounced word (קרי) is 111/106. Let's have the French mathematician Shlomo Belga pick up the story - in his paper (first published in the 1991 Proceedings of the 17th Canadian Congress of History and Philosophy of Mathematics, and recently updated), he gets rather excited about the whole gematria thing:
Another mathematician, Andrew Simoson also addresses this large bowl that is described in מלאכים א׳, the First Book of Kings and is often called Solomon's Sea. He doesn't buy the gematria, and wrote about it in The College Mathematics Journal.
A natural question with respect to this method is, why add, divide, and multiply the letters of the words? Perhaps an even more basic question is, why all the mystery in the first place? Furthermore, H. W. Guggenheimer, in his Mathematical Reviews...seriously doubts that the use of letters as numerals predates Alexandrian times; or if such is the case, the chronicler did not know the key. Moreover, even if this remarkable approximation to pi is more than coincidence, this explanation does not resolve the obvious measurement discrepancy - the 30-cubit circumference and the 10-cubit diameter. Finally, Deakin points out that if the deity truly is at work in this phenomenon of scripture revealing an accurate approximation ofpi... God would most surely have selected 355/113...as representative of pi...
Still, what stuck Simoson was that "...the chroniclers somehow decided that the diameter and girth measurements of Solomon's Sea were sufficiently striking to include in their narrative." (If you'd like another paper to read on this subject, try this one, published in B'Or Ha'Torah - the journal of "Science, Art & Modern Life in the Light of the Torah." You're welcome.)
PI IN THE TALMUD
The Talmud echoes the biblical value of pi in many places. For example:
תלמוד בבלי מסכת עירובין דף יד עמוד א
כל שיש בהיקפו שלשה טפחים יש בו רחב טפח. מנא הני מילי? - אמר רבי יוחנן, אמר קרא : ויעש את הים מוצק עשר באמה משפתו עד שפתו עגל סביב וחמש באמה קומתו וקו שלשים באמה יסב אתו סביב
"Whatever circle has a circumference of three tefachim must have a diameter of one tefach." The problem is that as we've already noted, this value of pi=3 is not accurate. It deviates from the true value of pi (3.1415...) by about 5%. Tosafot is bothered by this too.
תוספות, עירובין יד א
והאיכא משהו. משמע שהחשבון מצומצם וכן בפ"ק דב"ב (ד' יד:) גבי שני טפחים שנשתיירו בארון ששם ספר תורה מונח שהיא בהיקפה ששה טפחים ופריך כיון דלאמצעיתו נגלל נפיש ליה משני טפחים וכן בתר הכי דמשני בספר דעזרה לתחלתו נגלל ופריך אכתי תרי בתרי היכי יתיב משמע דמצומצם לגמרי וקשיא דאין החשבון מדוקדק לפי חכמי המדות
Tosafos can't find a good answer, and concludes "this is difficult, because the result [that pi=3] is not precise, as demonstrated by those who understand geometry."
PI IN THE RAMBAM
In his commentary on the Mishnah (Eruvin 1:5) Maimonides makes the following observation:
פירוש המשנה לרמב"ם מסכת עירובין פרק א משנה ה
צריך אתה לדעת שיחס קוטר העיגול להקפו בלתי ידוע, ואי אפשר לדבר עליו לעולם בדיוק, ואין זה חסרון ידיעה מצדנו כמו שחושבים הסכלים, אלא שדבר זה מצד טבעו בלתי נודע ואין במציאותו שיודע. אבל אפשר לשערו בקירוב, וכבר עשו מומחי המהנדסים בזה חבורים, כלומר לידיעת יחס הקוטר להקיפו בקירוב ואופני ההוכחה עליו. והקירוב שמשתמשים בו אנשי המדע הוא יחס אחד לשלשה ושביעית, שכל עיגול שקוטרו אמה אחת הרי יש בהקיפו שלש אמות ושביעית אמה בקירוב. וכיון שזה לא יושג לגמרי אלא בקירוב תפשו הם בחשבון גדול ואמרו כל שיש בהקיפו שלשה טפחים יש בו רוחב טפח, והסתפקו בזה בכל המדידות שהוצרכו להן בכל התורה.
...The ratio of the diameter to the circumference of a circle is not known and will never be known precisely. This is not due to a lack on our part (as some fools think), but this number [pi] cannot be known because of its nature, and it is not in our ability to ever know it precisely. But it may be approximated ...to three and one-seventh. So any circle with a diameter of one has a circumference of approximately three and one-seventh. But because this ratio is not precise and is only an approximation, they [the rabbis of the Mishnah and Talmud] used a more general value and said that any circle with a circumference of three has a diameter of one, and they used this value in all their Torah calculations.
So what are we to make of all this? Did the rabbis of the Talmud get pi wrong, or were they just approximating pi for ease of use? After considering evidence from elsewhere in the Mishnah (Ohalot 12:6 - I'll spare you the details), Judah Landa, in his book Torah and Science, has this to say:
We can only conclude that the rabbis of the Mishnah and Talmud, who lived about 2,000 years ago, believed that the value of pi was truly three. They did not use three merely for simplicity’s sake, nor did they think of three as an approximation for pi. On the other hand, rabbis who lived much later, such as the Rambam and Tosafot (who lived about 900 years ago), seem to be acutely aware of the gross innacuracies that results from using three for pi. Mathematicians have known that pi is greater than three for thousands of years. Archimedes, who lived about 2,200 years ago, narrowed the value of pi down to between 3 10/70 and 3 10/71 ! (Judah Landa. Torah and Science. Ktav Publishing House 1991. p.23.)
HAPPY BIRTHDAY, EINSTEIN
Today, March 14, is not only Pi Day. It is also the anniversary of the birthday of Albert Einstein, who was born on March 14, 1879. As I've noted elsewhere, Einstein was a prolific writer; one recent book (almost 600 pages long) claims to contain “roughly 1,600” Einstein quotes. It's hard to choose just one pithy quote of his on which to close. So here are two. Happy Pi Day. Happy birthday, Albert Einstein. And Shabbat Shalom from Talmudology
“As a human being, one has been endowed with just enough intelligence to be able to see clearly how utterly inadequate that intelligence is when confronted with what exists.”
“One thing I have learned in a long life: That all our science, measured against reality, is primitive and childlike — and yet it is the most precious thing we have.”

Talmudology on the Parsha, Vayakhel: How The Menorah Became the Emblem of The State of Israel
שמות 31:17–22
וַיַּ֥עַשׂ אֶת־הַמְּנֹרָ֖ה זָהָ֣ב טָה֑וֹר מִקְשָׁ֞ה עָשָׂ֤ה אֶת־הַמְּנֹרָה֙ יְרֵכָ֣הּ וְקָנָ֔הּ גְּבִיעֶ֛יהָ כַּפְתֹּרֶ֥יהָ וּפְרָחֶ֖יהָ מִמֶּ֥נָּה הָיֽוּ
וְשִׁשָּׁ֣ה קָנִ֔ים יֹצְאִ֖ים מִצִּדֶּ֑יהָ שְׁלֹשָׁ֣ה ׀ קְנֵ֣י מְנֹרָ֗ה מִצִּדָּהּ֙ הָֽאֶחָ֔ד וּשְׁלֹשָׁה֙ קְנֵ֣י מְנֹרָ֔ה מִצִּדָּ֖הּ הַשֵּׁנִֽי
שְׁלֹשָׁ֣ה גְ֠בִעִ֠ים מְֽשֻׁקָּדִ֞ים בַּקָּנֶ֣ה הָאֶחָד֮ כַּפְתֹּ֣ר וָפֶ֒רַח֒ וּשְׁלֹשָׁ֣ה גְבִעִ֗ים מְשֻׁקָּדִ֛ים בְּקָנֶ֥ה אֶחָ֖ד כַּפְתֹּ֣ר וָפָ֑רַח כֵּ֚ן לְשֵׁ֣שֶׁת הַקָּנִ֔ים הַיֹּצְאִ֖ים מִן־הַמְּנֹרָֽה׃
וּבַמְּנֹרָ֖ה אַרְבָּעָ֣ה גְבִעִ֑ים מְשֻׁ֨קָּדִ֔ים כַּפְתֹּרֶ֖יהָ וּפְרָחֶֽיהָ׃
וְכַפְתֹּ֡ר תַּ֩חַת֩ שְׁנֵ֨י הַקָּנִ֜ים מִמֶּ֗נָּה וְכַפְתֹּר֙ תַּ֣חַת שְׁנֵ֤י הַקָּנִים֙ מִמֶּ֔נָּה וְכַפְתֹּ֕ר תַּֽחַת־שְׁנֵ֥י הַקָּנִ֖ים מִמֶּ֑נָּה לְשֵׁ֙שֶׁת֙ הַקָּנִ֔ים הַיֹּצְאִ֖ים מִמֶּֽנָּה׃
כַּפְתֹּרֵיהֶ֥ם וּקְנֹתָ֖ם מִמֶּ֣נָּה הָי֑וּ כֻּלָּ֛הּ מִקְשָׁ֥ה אַחַ֖ת זָהָ֥ב טָהֽוֹר׃
And he made the Menorah of pure gold: of beaten work made he the Menorah; its shaft, and its branches, its bowls, its bulbs, and its flowers, were of the same piece: and six branches going out of its sides; three branches of the candlestick out of the one side of it, and three branches of the Menorah out of the other side of it: three bowls made after the fashion of almonds in one branch, a bulb and a flower; and three bowls made like almonds in another branch, a bulb and a flower: so throughout the six branches going out of the Menorah. And in the Menorah were four bowls made like almonds, its bulbs, and its flowers: and a bulb under two branches of the same, and a bulb under two branches of the same, and a bulb under two branches of the same, according to the six branches going out of it.. Their bulbs and their branches were of the same: all of it was one beaten work of pure gold.
The 1948 Search for an Emblem of the State of Israel
Immediately after the establishment of Israel, the Provisional State Council appointed a committee to create its official flag and national emblem. The committee publicized the search in a small newspaper announcement.
Among the few responses included one from the well known graphic designers Valish and Strosky. It featured the Menorah – the oldest Jewish symbol that can be positively identified, and that is first described in this week’s parsha. Their model of the Menorah was based on the one depicted on the Arch of Titus in Rome., and the row of seven gold stars is taken from Herzl’s own proposal for a flag. These seven stars symbolized the seven-hour workday that Herzl had envisioned for Jewish workers. In July 1948 the committee rejected Valish and Strosky’s proposal.
“One doesn’t chose a national emblem and flag every day”
Another newspaper notice was published, and this time there was more interest in the challenge. The committee received 131 entries, but only a few have been preserved in the State archives. Here are a some of them.
Graphic artists Gabriel and Maxim Shamir (whose proposal was ultimately selected), submitted a design. It depicted a stylized Menorah in modern form, specifically to break with tradition. Instead of curves there were six right angles rather than curves, and the heavy base was replaced with a tiny unsteady one, in an intentional dissociation from the traditional Jewish symbol.
In December 1948 the committee felt the Shamirs had the most promising design, but they wanted several changes, like adding the word “Israel” underneath. A month later, at the seventh meeting of the committee, they reevaluated the modern design they had requested. Being a committee, they changed their minds again, and asked that the Menorah on the Arch of Titus be used - the same Menorah that had been depicted on the earlier design of Valish and Storsky. Why? According to Professor Alec Meroshi, of the Open University in Israel, it was because of the symbolism:
“It was a visual metaphor for a concept that was popular at the time…just as the fall of the Jewish state in 70 CE found visual expression in the relief depicting Titus’ triumphal procession on the arch…so would the rebirth of the Jewish state and the termination of exile be symbolized by the return of the menorah to its homeland, if not to the Temple, then to the state of Israel that had just been established. The menorah was being removed from the arch, where it served as a symbol of defeat and degradation, and placed on the most honored spot of all – in the emblem of the State of Israel ” .
After some further minor revisions, the proposal was unanimously accepted on February 10th, 1949. The following day the provisional government published its “Proclamation of the Emblem of the State of Israel.”
Some of those first Israelis interpreted the design as as a victory for secular, socialist and democratic values over religious ones. But the combination of the Menorah and olive branches in the Shamir design actually had graphic precedents – from the vision of the prophet Zechariah, which is read on Shabbat Channukah and Pa’arshat Beha’alotecha:
זכריה ד
וַיֹּ֣אמֶר אֵלַ֔י מָ֥ה אַתָּ֖ה רֹאֶ֑ה וָאֹמַ֡ר רָאִ֣יתִי ׀ וְהִנֵּ֣ה מְנוֹרַת֩ זָהָ֨ב כֻּלָּ֜הּ וְגֻלָּ֣הּ עַל־רֹאשָׁ֗הּ וְשִׁבְעָ֤ה נֵרֹתֶ֙יהָ֙ עָלֶ֔יהָ שִׁבְעָ֤ה וְשִׁבְעָה֙ מֽוּצָק֔וֹת לַנֵּר֖וֹת אֲשֶׁ֥ר עַל־רֹאשָֽׁהּ׃ וּשְׁנַ֥יִם זֵיתִ֖ים עָלֶ֑יהָ אֶחָד֙ מִימִ֣ין הַגֻּלָּ֔ה וְאֶחָ֖ד עַל־שְׂמֹאלָֽהּ׃
And he said to me, What seest thou? And I said, I have looked, and behold a menorah all of gold, with a bowl upon the top of it, and seven lamps to it, and seven pipes to the seven lamps, which were upon the top of it: and there are two olive trees by it, one upon the right side of the bowl, and the other upon the left side of it.
Zechriah had seen the Menorah in the Second Temple which was completed in 521 C.E. This was apparently the same Menorah that was captured some 500 years later by Titus and carried off to Rome. And so the emblem of the State of Israel may well replicate the actual Menorah in the Bet Hamikdash, one that in turn is based on the description in this weeks’ parsha.
We don’t like it
Public reaction to the new emblem was swift and extreme; it was criticized by both the religious and the secular. On February 13, 1948, the very next day after the winning emblem had been announced, Gershon Schoken, editor of Haaretz wrote:
This proposal…is nothing but a horror from an aesthetic viewpoint…the execution is so vulgar and amateurish that no self-respecting commercial firm would even consider selecting it as its trademark…
Israel’s Chief Rabbi, Yitchak Halevi Herzog (and the grandfather of the current President of the State if Israel) was also annoyed.
What our government has done today is wrong…it has copied the depiction of the Menorah on the Arch of Titus, which was apparently the work of foreigners, and is not entirely in accordance with the sacred prescriptions…
It might be surprising to learn that the Emblem of Israel was initially controversial, but great decisions require compromise and understanding. What better message could there be for the State of Israel today?

Bava Metzia 2a ~ Garments, Game Theory and the Principal of Contested Sums
בבא מציעא ב,ב
שנים אוחזין בטלית ... זה אומר כולה שלי וזה אומר חציה שלי ...זה נוטל שלשה חלקים וזה נוטל רביע
Two hold a garment; ... one claims it all, the other claims half. ... Then the one is awarded 3⁄4, the other 1⁄4.
We open the new masechet of Bava Metzia with two people claiming ownership of a garment. One claims that it belongs entirely to her, and the other claims he owns half of the garment. In this case, the Mishnah rules that each swears under oath, and then the garment is divided with 3/4 awarded to one claimant and 1/4 to the other.
Rashi and the Principal of Contested Sums
In his explanation of our Mishnah, Rashi notes that the claimant to half the garment concedes that half belongs to the other claimant, so that the dispute revolves solely around the second half. Consequently, each of them receives half of this disputed half - or a quarter each.:
זה אומר חציה שלי. מודה הוא שהחצי של חבירו ואין דנין אלא על חציה הלכך זה האומר כולה שלי ישבע כו' כמשפט הראשון מה שהן דנין עליו נשבעין שניהם שאין לכל אחד בו פחות מחציו ונוטל כל אחד חציו
Now of course this is only one way that the garment could be divided between the two claimants. For example, it could be divided in proportion to the two claims, (2/3-1/3), or even split evenly (1/2-1/2). But instead, and as Rashi explained, the Mishnah ruled using the principal of contested sums. Which is where Robert Aumann comes in.
Game Theory from Israel's Nobel Prize Winner
We have met Robert Aumann before, when we reviewed Israel's glorious winners of the Nobel Prize. For those who need reminding, Aumanm, from the Hebrew University, won the 2005 Nobel Prize in Economics. His work was on conflict, cooperation, and game theory (yes, the same kind of game theory made famous by the late John Nash, portrayed in A Beautiful Mind). Aumann worked on the dynamics of arms control negotiations, and developed a theory of repeated games in which one party has incomplete information. The Royal Swedish Academy of Sciences noted that this theory is now "the common framework for analysis of long-run cooperation in the social science." The kippah-wearing professor opened his speech at the Nobel Prize banquet with the following words (which were met with cries of אמן from some members of the audience):
ברוך אתה ה׳ אלוקנו מלך העולם הטוב והמיטב
If you haven't already seen it, take the time to watch the four-minute video of his acceptance speech. It should be required viewing for every Jewish high school student (and their teachers).
Where was I? Oh, yes. Contested sums. In 1985, twenty years before receiving his Nobel Prize, Aumann described the theoretic underpinnings of today's Mishnah, as part of a larger discussion about bankruptcy. His paper, published in the Journal of Economic Theory, is heavy on mathematical notations and light on explanations for non-mathematicians (like me). Fortunately he later published a paper that is much easier to read and which covers the same material. The second paper appeared in the Research Bulletin Series on Jewish Law and Economics, published by Bar-Ilan University in June 2002. "Half the garment" wrote the professor, "is not contested: There is general agreement that it belongs to the person who claimed it all. Hence, first of all, that half is given to him. The other half, which is claimed by both, is then divided equally between the claimants, each receiving one-quarter of the garment." Here is how Aumann visualizes it:
There is another example of this from the Tosefta, a supplement to the Mishnah and contemporary with it. In this new case, one person claims the entire garment, and one claims only one third of it. In this case, the first person gets 5/6 and the second gets 1/6.
Aumann calls this principal the "Contested Garment Consistent." It turns out that this principal is found in other contested divisions, like a case in Ketuvot 93a, in which a man dies, leaving debts totaling more than his estate. The Mishnah explains how the estate should be divided up among his three wives, each of who has a claim. And it uses the same principal as the one found in today's Mishnah: the Contested Garment Consistent.
משנה, כתובות צג, א
מי שהיה נשוי שלש נשים ומת כתובתה של זו מנה ושל זו מאתים ושל זו שלש מאות ואין שם אלא מנה חולקין בשוה
היו שם מאתים של מנה נוטלת חמשים של מאתים ושל שלש מאות שלשה שלשה של זהב
היו שם שלש מאות של מנה נוטלת חמשים ושל מאתים מנה ושל שלש מאות ששה של זהב
If a man who was married to three wives died, and the kethubah of one was a maneh (one hundred zuz), of the other two hundred zuz, and of the third three hundred zuz, and the estate was worth only one maneh (one hundred zuz), they divide it equally.
If the estate was worth two hundred zuz, the claimant with the kesuva of the maneh receives fifty zuz, while the and the claimants of the two hundred and the three hundred zuz each receive three gold denarii (worth seventy-five zuz).
If the estate was worth three hundred zuz, the claimant of the maneh receives fifty zuz, the claimant of the two hundred zuz receives a maneh (one hundred zuz) and the the claimant of the three hundred zuz receives six gold denarii (worth one hundred and fifty zuz)…
Aumann likes to think of it this way:
Here is how he explains what is going on in the Mishnah in Ketubot.
We’ll call the creditor with the 100-dinar claim “Ketura,” the one with the 200, “Hagar” and the one with the 300, “Sara.” Let’s assume, to begin with, that the estate is 200. As per [the table above], Ketura gets 50 and Hagar 75 – together 125. On the principle of equal division of the contested sum, the 125 gotten by Hagar and Ketura together should be divided between them in keeping with this principle. ... In other words, the Mishna’s distribution reflects a division of the sum that Hagar and Ketura receive together according to the principle of equal division of the contested sum...
The division of the estate among the three creditors is such that any two of them divide the sum they together receive, according to the principle of equal division of the contested sum. This precisely is the method of division laid down in the Mishna in Bava Metzia that deals with the contested garment.
There's a lot more to the paper, including an interesting proof of the principal using fluids poured into cups of different sizes. But I prefer to focus on another aspect of the paper. Prof. Aumann notes that, in addition to its own internal logic, the underlying principal of contested sums fits in well with other talmudic passages.
The reader may ask, isn’t it presumptuous for us to think that we succeeded in unraveling the mysteries of this Talmudic passage, when so many generations of scholars before us failed? To this, gentle reader, we respond that the scholars who studied and wrote about this passage over the course of almost two millennia were indeed much wiser and more learned than we. But we brought to bear a tool that was not available to them: the modern mathematical theory of games.
The actual sequence of events was that we first discovered that the Mishnaic divisions are implicit in certain sophisticated formulas of modern game theory. Not believing that the sages of the Talmud could possibly have been aware of these complex mathematical tools, we sought, and eventually found, a conceptual basis for these tools: the principle of consistency. Of this, the sages could have been, and presumably were, aware. It in itself is sufficient to yield the Mishnaic divisions; and it is this principle that we describe below, bypassing the intermediate step – the game theory.
It’s like “Alice in Wonderland.” The game theory provides the key to the garden, which Alice had such great difficulty in obtaining. Once in the garden, though, Alice can discard the key; the garden can be enjoyed without it.
What a wonderful analogy. Game theory is the key to entering the garden, a key which might not have been available to earlier generations who learned the Talmud. How lucky we are.