Today we discuss maths (as it is called in the UK, Ireland and Australia,) or math (as it is called in the US and Canada). We will focus on that most magical of numbers, pi, also known as π. On today’s page of Talmud we find this theorem:
תלמוד בבלי מסכת בבא בתרא יד, א
כֹּל שֶׁיֵּשׁ בְּהֶקֵּיפוֹ שְׁלֹשָׁה טְפָחִים
Elsewhere, the Talmud determined the that value of π is 3 from a verse in the Book of Kings:
מלכים א פרק ז פסוק כג
ויעש את הים מוצק עשר באמה משפתו עד שפתו עגל סביב וחמש באמה קומתו וקוה שלשים באמה יסב אתו סביב
And he made a molten sea, ten amot from one brim to the other: it was round, and its height was five amot, and a circumference of thirty amot circled it.
The Talmud in Eruvin uses this verse to teach a general mathematical principle:
כל שיש בהיקפו שלשה טפחים יש בו רחב טפח. מנא הני מילי? - אמר רבי יוחנן, אמר קרא : ויעש את הים מוצק עשר באמה משפתו עד שפתו עגל סביב וחמש באמה קומתו וקו שלשים באמה יסב אתו סביב
"Whatever circle has a circumference of three tefachim must have a diameter of one tefach."
So one of the vessels in the Temple of Solomon was ten amot in diameter and 30 amot in circumference. Since π is the ratio of the circumference of a circle to its diameter (π=C/d), π in the Book of Kings is 30/10=3. Three - no more and no less.
But PI is more than three
However, this value of π =3 is not accurate. It deviates from the true value of π (3.1415...) by about 5%. Tosafot in Eruvin is bothered by this too.
תוספות, עירובין יד א
והאיכא משהו. משמע שהחשבון מצומצם וכן בפ"ק דב"ב (ד' יד:) גבי שני טפחים שנשתיירו בארון ששם ספר תורה מונח שהיא בהיקפה ששה טפחים ופריך כיון דלאמצעיתו נגלל נפיש ליה משני טפחים וכן בתר הכי דמשני בספר דעזרה לתחלתו נגלל ופריך אכתי תרי בתרי היכי יתיב משמע דמצומצם לגמרי וקשיא דאין החשבון מדוקדק לפי חכמי המדות
Tosafot opens the objection with these words: “But [pi] is a little more [than 3]. Which means that the value [of pi] is rounded down” Tosafot can't find a good answer to this obvious problem, and concludes "this is difficult, because the result [that pi=3] is not precise, as demonstrated by those who understand geometry."
PI IN THE RAMBAM
In his commentary on the Mishnah on which today’s discussion is based, (Eruvin 1:5) Maimonides makes the following observation:
פירוש המשנה לרמב"ם מסכת עירובין פרק א משנה ה
צריך אתה לדעת שיחס קוטר העיגול להקפו בלתי ידוע, ואי אפשר לדבר עליו לעולם בדיוק, ואין זה חסרון ידיעה מצדנו כמו שחושבים הסכלים, אלא שדבר זה מצד טבעו בלתי נודע ואין במציאותו שיודע. אבל אפשר לשערו בקירוב, וכבר עשו מומחי המהנדסים בזה חבורים, כלומר לידיעת יחס הקוטר להקיפו בקירוב ואופני ההוכחה עליו. והקירוב שמשתמשים בו אנשי המדע הוא יחס אחד לשלשה ושביעית, שכל עיגול שקוטרו אמה אחת הרי יש בהקיפו שלש אמות ושביעית אמה בקירוב. וכיון שזה לא יושג לגמרי אלא בקירוב תפשו הם בחשבון גדול ואמרו כל שיש בהקיפו שלשה טפחים יש בו רוחב טפח, והסתפקו בזה בכל המדידות שהוצרכו להן בכל התורה
...The ratio of the diameter to the circumference of a circle is not known and will never be known precisely. This is not due to a lack on our part (as some fools think), but this number [pi] cannot be known because of its nature, and it is not in our ability to ever know it precisely. But it may be approximated ...to three and one-seventh. So any circle with a diameter of one has a circumference of approximately three and one-seventh. But because this ratio is not precise and is only an approximation, they [the rabbis of the Mishnah and Talmud] used a more general value and said that any circle with a circumference of three has a diameter of one, and they used this value in all their Torah calculations.
Is the Value of Pi hidden in the Bible?
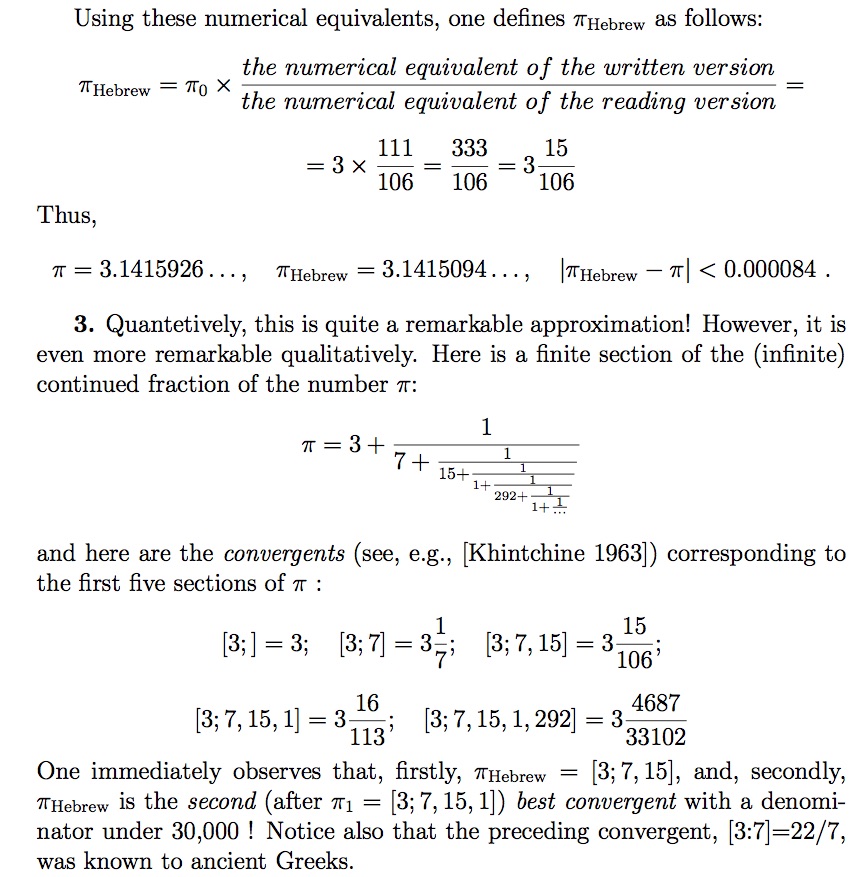
There are lots of papers on the value of pi in the the Bible. Many of them mention an observation that seems to have been incorrectly attributed to the Vilna Gaon. The verse we cited from מלאכים א׳ spells the word for line as קוה, but it is pronounced as though it were written קו. (In דברי הימים ב׳ (II Chronicles 4:2) the identical verse spells the word for line as קו.) The ratio of the numerical value (gematria) of the written word (כתיב) to the pronounced word (קרי) is 111/106. Let's have the French mathematician Shlomo Belga pick up the story - in his paper (first published in the 1991 Proceedings of the 17th Canadian Congress of History and Philosophy of Mathematics, and recently updated), he gets rather excited about the whole gematria thing:
A mathematician called Andrew Simoson also addresses this large tub that is described in מלאכים א׳ and is often called Solomon's Sea. He doesn't buy the gematria, and wrote about it in The College Mathematics Journal.
A natural question with respect to this method is, why add, divide, and multiply the letters of the words? Perhaps an even more basic question is, why all the mystery in the first place? Furthermore, H. W. Guggenheimer, in his Mathematical Reviews...seriously doubts that the use of letters as numerals predates Alexandrian times; or if such is the case, the chronicler did not know the key. Moreover, even if this remarkable approximation to pi is more than coincidence, this explanation does not resolve the obvious measurement discrepancy - the 30-cubit circumference and the 10-cubit diameter. Finally, Deakin points out that if the deity truly is at work in this phenomenon of scripture revealing an accurate approximation of pi... God would most surely have selected 355/113...as representative of pi...
Still, what stuck Simoson was that "...the chroniclers somehow decided that the diameter and girth measurements of Solomon's Sea were sufficiently striking to include in their narrative." (If you'd like another paper to read on this subject, try this one, published in B'Or Ha'Torah - the journal of "Science, Art & Modern Life in the Light of the Torah." You're welcome.)
Did the rabbis of the Talmud get π wrong?
So what are we to make of all this? Did the rabbis of the Talmud get π wrong, or were they just approximating π for ease of use? After considering evidence from elsewhere in the Mishnah (Ohalot 12:6 - I'll spare you the details), Judah Landa, in his book Torah and Science, has this to say:
We can only conclude that the rabbis of the Mishnah and Talmud, who lived about 2,000 years ago, believed that the value of pi was truly three. They did not use three merely for simplicity’s sake, nor did they think of three as an approximation for pi. On the other hand, rabbis who lived much later, such as the Rambam and Tosafot (who lived about 900 years ago), seem to be acutely aware of the gross innacuracies that results from using three for pi. Mathematicians have known that pi is greater than three for thousands of years. Archimedes, who lived about 2,200 years ago, narrowed the value of pi down to between 3 10/70 and 3 10/71 ! (Judah Landa. Torah and Science. Ktav Publishing House 1991. p.23.)
Still, don't be too hard on the rabbis of the Talmud. The rule that the circumference of an object is three times its diameter is pretty close to being correct, and is usually a good enough approximation. But it is not accurate, and never will be.